Light
Reflection
Characteristics of Reflected Images:
- Magnification
- Attitude (upright or inverted)
- Kind (real or virtual)
- Position (opposite or same side as object)
Laws of Reflection:
- In plane (flat) mirrors, the incident angle is equal to the reflected angle.
- The two most important types of curved mirrors are converging (concave) and diverging (convex).
- You can find where an image will be projected and the magnification by either the mirror and magnification equations or by drawing a scale ray diagram (pictured below).
There are three main rays that are drawn when creating a diagram:
1. Comes parallel to principal axis from object, reflects through the focus.
2. Goes from object through focus, reflects out parallel.
3. Goes from object through centre, reflects straight back out (not pictured).
With a diverging mirror, the focal length is on the other side of the mirror than it is with a converging mirror.
Lab Summary- Curved Mirrors
This lab focused on the characteristics of the images formed by curved mirrors and using the mirror and magnification equations as well as ray diagrams.
These are the characteristics for a converging mirror according to where the object is placed:
Beyond center of curvature
1. Magnification- smaller
2. Attitude- inverted
3. Kind- real image
4. Position- same side
At the center of curvature
1. Same side
2. Inverted
3. Real
4. Same side
Between the focal point and radius of curvature
1. Larger
2. Inverted
3. Real
4. Same side
At the focal point
*No image produced.
Inside the focal point
1. Larger
2. Upright
3. Virtual
4. Opposite side
For a diverging mirror:
All locations in front of mirror
1. Smaller
2. Erect
3. Virtual
4. Opposite side
Formulas
Magnification Equation
hi = height of image
ho= height of object
di = distance of image
do= distance of object
f = focal length
di = distance of image
do= distance of object
* For a diverging mirror, the focal length
must be negative
Refraction
Index of refraction- a number that indicates the amount of refraction when light changes medium.
Snell’s Law
This lab was about the mathematical relationship between the angles created by the incident and refracted rays when a ray of light changes substance. We used a piece of glass as our new substance and measured the angle at which objects placed on the other side appeared. These angles are always measured to the normal line. Our observations were correspondent with Snell’s Law, that sine of the angle of incidence divided by sine of the angle of refraction will give you a constant, the index of refraction (n).
Critical Angle and Total Internal Reflection
Critical angle- the incident angle at which the refracted angle becomes 90º
Total internal reflection- when the incident angle is greater than the critical angle
- When light travels from less dense to more dense the light bends towards the normal line.
- When light travels from more to less dense the light bends further away from the normal line .

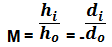



No comments:
Post a Comment